Straight Line Graphs - League of Learning

Straight Line Graphs (y = mx + c)

Finding Equations of Straight Lines

Why do we want to know about straight lines and their equations?

Straight Line Graphs (Linear Graphs) have lots of uses in mathematics – one use is in navigation
We may want to know the equation of a straight line so we can program it into a computer that will plot the line on a screen, along with several others, to make shapes and graphics

How do we find the equation of a straight line?

The general equation of a straight line is $y = m x + c$ where;
- $m$ is the gradient,
- $c$ is the $y$ -axis intercept (or simply, the $y$ -intercept)
To find the equation of a straight line you need TWO things:
1. the gradient, $m$ , which you can put straight into $y = m x + c$
  - get this from the question directly, or from two points using $\frac{rise}{run}$ or the gradient formula
2. any point on the line- substitute this point into $y = m x + c$ (as you already know $m$ ) and solve to find $c$
  - if given two points which you used to find the gradient, just choose either one of them for the point to find $c$
You may be asked to give the equation in the form $a x + b y + c = 0$
(especially if $m$ is a fraction)
If in doubt, SKETCH IT!

What if the line is not in the form y=mx+c?

A line could be given in the form $a x + b y + c = 0$
- It is harder to identify the gradient and intercept in this form
We can rearrange the equation into $y = m x + c$ , so it is easier to identify the gradient and intercept
- - $a x + b y + c = 0$
- Subtract $a x$ from both sides
  - $b y + c = - a x$
- Subtract $c$ from both sides
  - $b y = - a x - c$
- Divide both sides by $b$
  - $y = - \frac{a x}{c} - \frac{c}{b}$
  - $y = - \frac{a}{c} x - \frac{c}{b}$
- In this case, the gradient is $- \frac{a}{c}$ and the $y$ -intercept is $- \frac{c}{b}$

Worked example

(a)

Find the equation of the straight line with gradient 3 that passes through (5, 4).

We know that the gradient is 3 so the line takes the form

$y = 3 x + c$

To find the value of c, substitute (5, 4) into the equation

$4 = 3 (5) + c 4 = 15 + c c = - 11$

Replace c with −11 to complete the equation of the line

y = 3x − 11

(b)

Find the equation of the straight line that passes through (-2, 6) and (8, 1).

You may find it helpful to sketch the information given

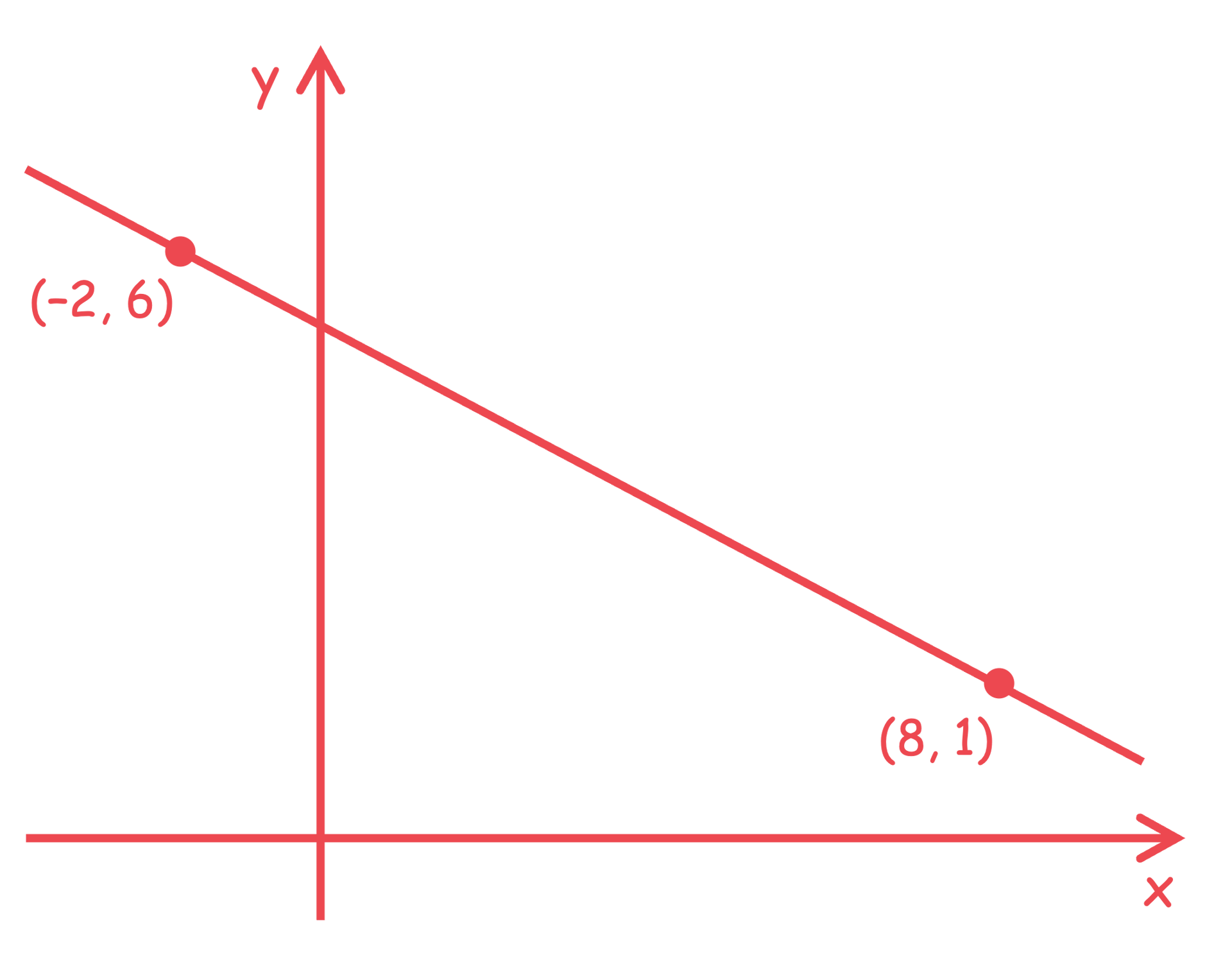
XXg8k5ry_2-13-1-finding-equations-of-straight-lines

First find m, the gradient

\begin{array}{rcl} m & = & \frac{6 - 1}{- 2 - 8} \\ = & \frac{5}{- 10} \\ = & - \frac{1}{2} \end{array}

We know that the line takes the form

\begin{array}{rcl} y & = & - \frac{1}{2} x + c \end{array}

To find the value of c, substitute either of the given points into this equation. Here we will pick (8, 1) as it is doesn't contain negative numbers so is easier to work with

$1 = - \frac{1}{2} (8) + c 1 = - 4 + c c = 5$

Replace c with −11 to complete the equation of the line

$y = - \frac{1}{2} x + 5$

We can check against our sketch that this equation looks correct- it has a negative gradient and it crosses the y-axis between 1 and 6

Drawing Linear Graphs

How do we draw the graph of a straight line from an equation?

Before you start trying to draw a straight line, make sure you understand how to find the equation of a straight line – that will help you understand this
How we draw a straight line depends on what form the equation is given in
There are two main forms you might see:
y = mx + c and ax + by = c
Different ways of drawing the graph of a straight line:

From the form y = mx + c
(you might be able to rearrange to this form easily)
plot c on the y-axis
go 1 across, m up (and repeat until you can draw the line)
From ax + by = c
put x = 0 to find y-axis intercept
put y = 0 to find x-axis intercept
(You may prefer to rearrange to y = mx + c and use above method)

Exam Tip

It might be easier just to plot ANY two points on the line (a third one as a check is not a bad idea either)
or use the TABLE function on your calculator.

Worked example

On the axes below, draw the graphs of $y = 3 x - 1$ and $3 x + 5 y = 15$ .

For $y = 3 x - 1$ , first plot c, which is (0, −1)

Then, as m = 3, $\frac{rise}{run} = \frac{3}{1}$ . So plot a point 3 up and 1 right. Repeat at least once more and then join the points with a straight line. Extend the line to the edges of the grid.

The steps for $3 x + 5 y = 15$ are the same, but first we need to rearrange into the form y = mx + c

$\begin{array}{rcl} 5 y & = & - 3 x + 15 \\ y & = & - \frac{3}{5} x + 3 \end{array}$

Now we can plot c, which is (0, 3)

For the gradient, $\frac{rise}{run} = \frac{- 3}{5}$ . So plot a point 3 down and 5 right. There isn't space on the grid to repeat this so join the points with a straight line. Extend the line to the edges of the grid. The answer is shown above